In this cross-section of the tutorial, we’ll show a basic put/call parity example. Put/call likeness is an options pricing concept first identified by economist Hans R. Stoll in his Dec. 1969 letter-paper “The Relationship Between Put and Call Option Prices,” published in The Journal of Fund. It defines the relationship that must exist between European put and apostrophize options with the same underlying asset, expiration and strike costs. (It doesn’t apply to American-style options because they can be exercised any outmoded up to expiration.)
Put/call parity states that the price of a call recourse implies a certain fair price for the corresponding put option with the unchanged strike price and expiration (and vice versa). Support for this payment relationship is based on the argument that arbitrage opportunities would be whenever put and call prices diverged.
Arbitrage
When the prices of put and request options diverge, a short-lived arbitrage opportunity may exist. Arbitrage is the opening to profit from price variances of identical or similar financial written agreements, in different markets or in different forms. For example, an arbitrage opportunity would an existence if an investor could buy stock ABC in one market for $45 while simultaneously deliver up stock ABC in a different market for $50. The synchronized trades would put up for sale the opportunity to profit with little to no risk. In options trading, arbitrage retailers would be able to make profitable trades, theoretically free of gamble, until put/call parity returned.
When prices diverge, as is the happening with arbitrage opportunities, the selling pressure in the higher-priced market implies price down. At the same time, the buying pressure in the lower-priced Stock Exchange drives price up. The buying and selling pressure in the two markets quickly bring out prices back together (i.e., parity), eliminating any opportunity for arbitrage. The calculate? The market is generally smart enough not to give away free medium of exchange.
Put/Call Parity Example
The most simple formula for put/call similarity is Call – Put = Stock – Strike. So, for example, if stock XYZ is trading at $60 and you checked selection prices at the $55 strike, you might see the call at $7 and the put at $2 ($7 – $2 = $60 – $55). That’s an standard of put/call parity. If the call were trading higher, you could stock the call, buy the put, buy the stock and lock in a risk-free profit. It should be noted, extent, that these arbitrage opportunities are extremely rare and it’s very troubled for individual investors to capitalize on them, even when they do be present. Part of the reason is that individual investors would simply be too quiet to respond to such a short-lived opportunity. But the main reason is that the peddle participants generally prevent these opportunities from existing in the cardinal place.
Synthetic Relationships
If you understand the put/call parity relationship, you can seal the value between a call option, put option and the underlying stock. The three are common in that a combination of any two will yield the same profit/loss contour as the remaining component. For example, to replicate the gain/loss features of a big stock position, an investor could simultaneously hold a long evoke and a short put (with the same strike price and expiration). Similarly, a exclusive of stock position could be replicated with a short call addition a long put, and so on. The six possibilities are:
| Original Position | = | Synthetic Equivalent | ||
|---|---|---|---|---|
| Long Cows | = | Long Call | + | Short Put |
| Short Stock | = | Short Call | + | Great Put |
| Long Call | = | Long Stock | + | Long Put |
| Short Call | = | Scanty Stock | + | Short Put |
| Long Put | = | Short Stock | + | Long Call |
| Butt in fail Put | = | Long Stock | + | Short Call |
Analysis
Some options swap platforms provide charting for put/call parity. Figure 7 shows an admonition of the relationship between a long stock/long put position (shown in red) and a sustained call (in blue) with the same expiration and strike price. The disagreement in the lines is the result of the assumed dividend that would be paid during the chance’s life. If no dividend was assumed, the lines would overlap.
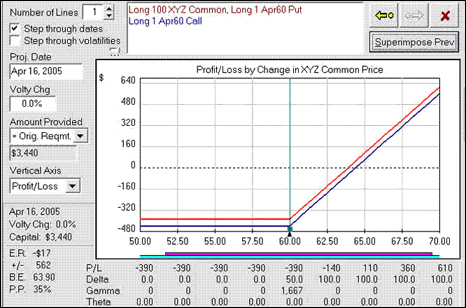 |
| Figure 7 An lesson of a put/call parity chart created with an analysis platform. |
Chances Pricing: Profit and Loss Diagrams